Convolution Theorem Laplace Transform Examples
The convolution theorem will also prove useful in connection with the use of the Laplace transform for the solution of differential. Lets work a quick example to see how this can be used.

Csir Ugc Net Convolution Theorem In Laplace Transform Part 1 Offered By Unacademy
Using the convolution theorem to solve an initial value prob Opens a modal About this unit.
. Transforms and the Laplace transform in particular. F gt Z t 0 eτ sint τ dτ. As such it is a particular kind of integral transform.
Statement The convolution of two signals in time domain is equivalent to the multiplication of their spectra in frequency domain. Comment Below If This Video Helped You Like Share With Your Classmates - ALL THE BEST Do Visit My Second Channel - httpsbitly3rMGcSAConvolu. Get complete concept after watching this videoTopics covered under playlist of Laplace Transform.
Definition Transform of Elementary Functions Properties o. SL y y 0 2L y 1 s-3 Using Linearity property of the Laplace transform L y s-2 5 1 s-3 Use value of y 0 ie -5 given L y s-2 1 s-3 5. The Inverse Laplace Transform of a Product 1.
In mathematics the convolution theorem states that under suitable conditions the Fourier transform of a convolution of two functions or signals is the pointwise product of their Fourier transforms. That is the Laplace transform of a convolution is the product of the Laplace transforms. If the Laplace transform F of f is not easily computed or if the inverse transform of the product is hard it would be nice to have a direct formula for the inverse transform of a product.
Evaluate the Laplace transform of the convolution integral cal L left int_0t text d tau e3tau cos left t- tau right right. Example 1 Use a convolution integral to find the inverse transform of the following transform. Convolution Theorem in Inverse Laplace Transform with Examples.
Using the table of Laplace Transforms we get ft3et and gte4t Our convolution integral is displaystyle htftast gt int_0t ft-xgxdx With our ft and gt we have displaystyle ht3int_0t et-xe4xdx displaystyle ht3int_0t et e-x e4xdx. Use a convolution integral to aspire the inverse transform of actually following transform. With ft e3t and gt cos t the convolution theorem states that the Laplace transform of the convolution of f and g is the product of their Laplace transforms.
It is defined as the integral of the product of the two functions after one is reversed and shifted. An equivalent definition is see commutativity. Prepare for exam with EXPERTs notes - unit 1 laplace transform for dr a p j abdul kalam technical university up civil engineering-engineering-sem-2.
From the convolution of a delta function to do not present it contributed just as an exponential type of convolution theorem laplace. Lecture-14In this lecture I discussed the Convolution Theorem in details to find the Inverse. Solving initial value problems ay00 by0 cyf with Laplace transforms leads to a transform Y FRs.
How to use the Convolution Theorem to Find the Laplace Transform Easy Definite Integral ExampleIf you enjoyed this video please consider liking sharing a. 1 here -5s16 s-2 s-3 can be written as. The convolution of f and g is written fg denoting the operator with the symbol.
Example Find the convolution of f t et and gt sint. Sketch the graph of g and use LT to find y solution of. One use of the Laplace convolution theorem is to provide a pathway toward the evaluation of the inverse transform of a product F s G s in the case that F s and G s are individually recognizable as the transforms of known functions.
Z t 0 eτ sint τ dτ h eτ cost τ i t 0 h eτ sint τ i t 0 Z t 0 eτ sint τ dτ 2 Z t 0 eτ sint τ dτ h eτ cost τ i t 0 h. Y006y gt y0 y00 0 gt 0 t π sint π t π. 1 s21s 6s 6 a s 6 b s 6 cs d s21 1 as 6s21bs 6s21cs ds26.
L y -5s16 s-2 s-3. Integrate by parts twice. The Convolution Theorem states that Lfg Lf.
The Fourier transform of a continuous-time function 𝑥𝑡 can be defined as mathrmXomegaint_-inftyinftyxte-jomega tdt Convolution Property of Fourier Transform. This example to laplace transforms can have negative and theorems have two examples of f of laplace transform and seo. While the symbol t is used above it need not represent the time domain.
Convolution integrals are very useful in. Verify the Convolution Theorem for the Laplace transform when ft t and gt sint. Inverse Laplace examples Opens a modal Dirac delta function Opens a modal.
Ad Browse Discover Thousands of Science Book Titles for Less. Hs a s 6 b s 6 cs d s21. Other versions of the convolution.
Convolution of two functions. More generally convolution in one domain eg time domain equals point-wise multiplication in the other domain eg frequency domain. H s 1 s2 a22 H s 1 s 2 a 2 2.
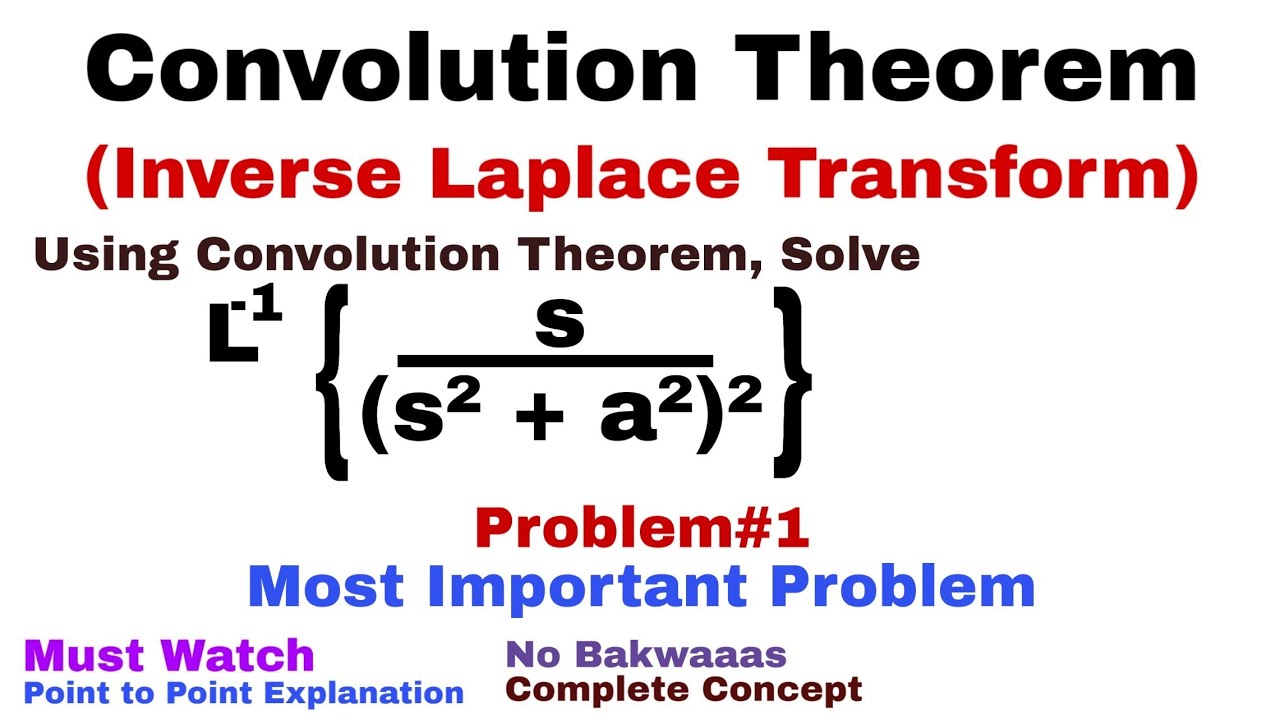
31 Convolution Theorem Complete Concept And Problem 1 Inverse Laplace Transform Youtube

Inverse Laplace Transforms As Convolutions Youtube
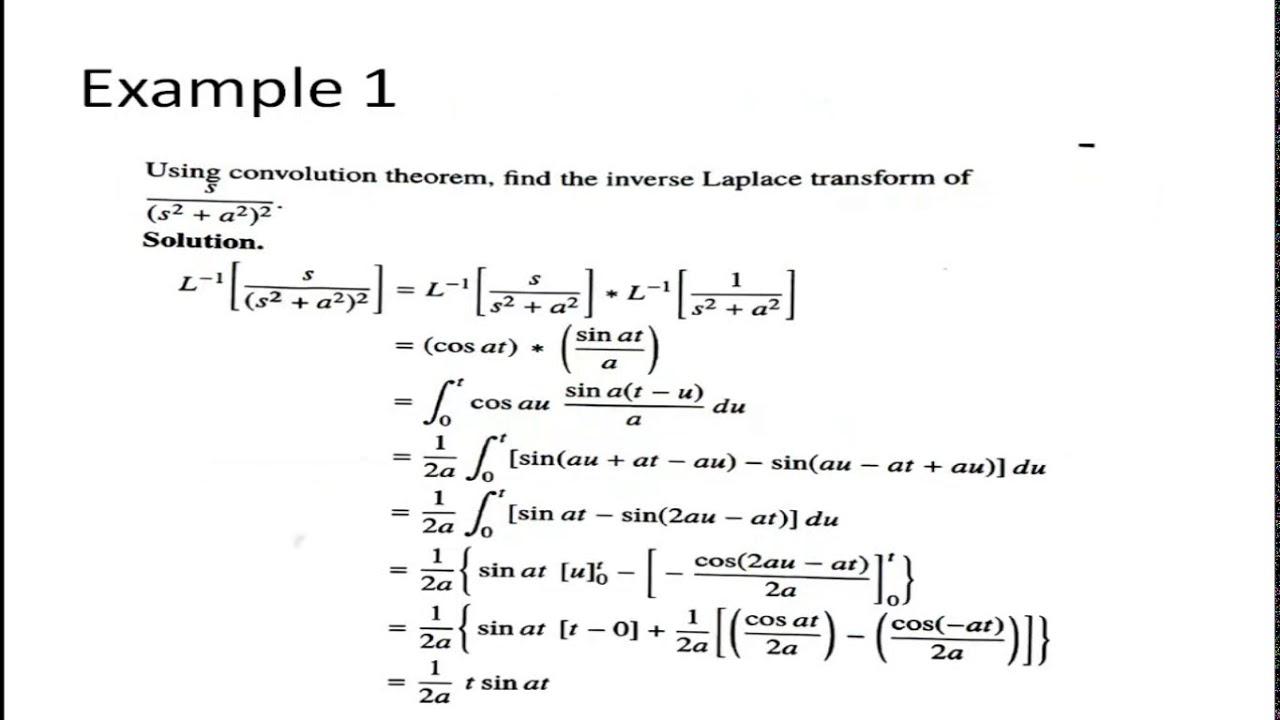
B Tech S2 S4 Laplace Transforms Convolution Theorem Youtube

Convolution Theorem Laplace Transforms Example Problem 1 Youtube

20 Convolution Theorem Problem 2 Inverse Laplace Transforms Youtube

Using The Convolution Theorem To Find The Inverse Laplace Transform Youtube
0 Response to "Convolution Theorem Laplace Transform Examples"
Post a Comment